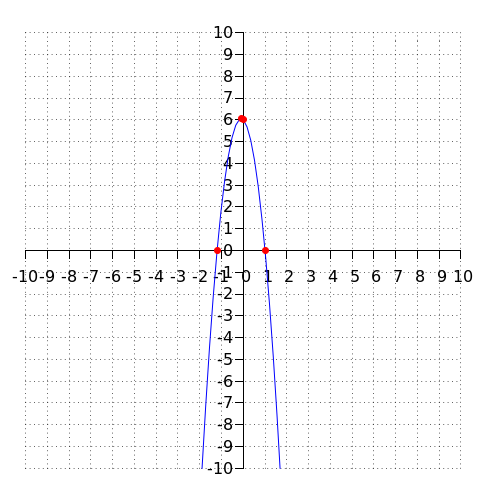
The red dots are the four dots from the question. These are calculated as follows:
The formula is already written in the form of #a \cdot x^2+b \cdot x +c# with #a =-2#, #b=5# and #c=6#. Seeing as #a<0# the graph is a parabola that opens downward.

The intersection with the #y#-axis is equal to the value of the constant in the quadratic formula, which is equal to #6#. That means that the coordinates of the intersection point with the #y#-axis are #\rv{0,6}#.

The #x#-value of the vertex is given by #x=-\dfrac{b}{2 \cdot a}# and is equal to:
\[\begin{array}{rclrl}
x&=& -\dfrac{5}{2 \cdot -2} &&\phantom{xxx}\blue{\text{formula entered}}\\
&=& {{5}\over{4}} &&\phantom{xxx}\blue{\text{simplified}}\\
\end{array}\]
The #y#-value of the vertex is calculated by entering #x={{5}\over{4}}# in the formula. Which gives:
\[\begin{array}{rclrl}
y&=& -2 \cdot {{5}\over{4}}^2 +5 \cdot {{5}\over{4}} +6
&&\phantom{xxx}\blue{\text{formula entered}}\\
&=& {{73}\over{8}} &&\phantom{xxx}\blue{\text{calculated}}\\
\end{array}\]
The coordinates of the vertex are: #\rv{{{5}\over{4}},{{73}\over{8}}}#. To draw the point in the graph, we have to write the coordinates as decimal numbers (rounded to 1 decimal). That gives: #\rv{1.2,9.1}#.

The intersections with the #x#-axis are the points that correspond to #y=0#.
\[\begin{array}{rcl}
-2\cdot x^2+5\cdot x+6 &=& 0 \\&&\phantom{xxx}\blue{\text{the equation that should be calculated}}\\
x=\dfrac{-{5}-\sqrt{5^2-4 \cdot -2 \cdot 6}}{2 \cdot -2} &\vee& x=\dfrac{-{5}+\sqrt{5^2-4 \cdot -2 \cdot 6}}{2 \cdot -2} \\&&\phantom{xxx}\blue{\text{quadratic formula entered}}\\
x={{5-\sqrt{73}}\over{4}} &\vee& x={{\sqrt{73}+5}\over{4}} \\&&\phantom{xxx}\blue{\text{calculated}}\\
\end{array}\]
The coordinates of the intersections with the #x#-axis are: #\rv{{{5-\sqrt{73}}\over{4}},0}# and #\rv{{{\sqrt{73}+5}\over{4}},0}#. To draw the point in the graph, we have to write the coordinates as decimal numbers (rounded to 1 decimal). That gives:#\rv{-0.9,0}# and #\rv{3.4,0}#.

The four points in the graph are: #\rv{0,6}#, #\rv{{{5}\over{4}},{{73}\over{8}}}#, #\rv{{{5-\sqrt{73}}\over{4}},0}# and #\rv{{{\sqrt{73}+5}\over{4}},0}#.